The Ricci Tensor: A Complete Guide With Examples

The Ricci tensor is an important mathematical object used in differential geometry that also shows up a lot in the general theory of relativity, among other things. But what does it actually represent?

The Ricci tensor represents how a volume in a curved space differs from a volume in Euclidean space. In particular, the Ricci tensor measures how a volume between geodesics changes due to curvature. In general relativity, the Ricci tensor represents volume changes due to gravitational tides.

Now, what does all of the above stuff actually mean? In this article I’ll be going over exactly that. We’ll also be looking at some properties of the Ricci tensor as well as practical examples of some commonly used Ricci tensors.

In case you’d want an ad-free PDF version of this article (an my other general relativity articles), you’ll find it here, available as part of my full General Relativity Bundle.

What Are Tensors, Intuitively?

Essentially, tensors are mathematical objects that are used in many areas of physics (in general relativity, for example, because they have some very useful transformation properties) and also in many areas of mathematics (for example, differential geometry).

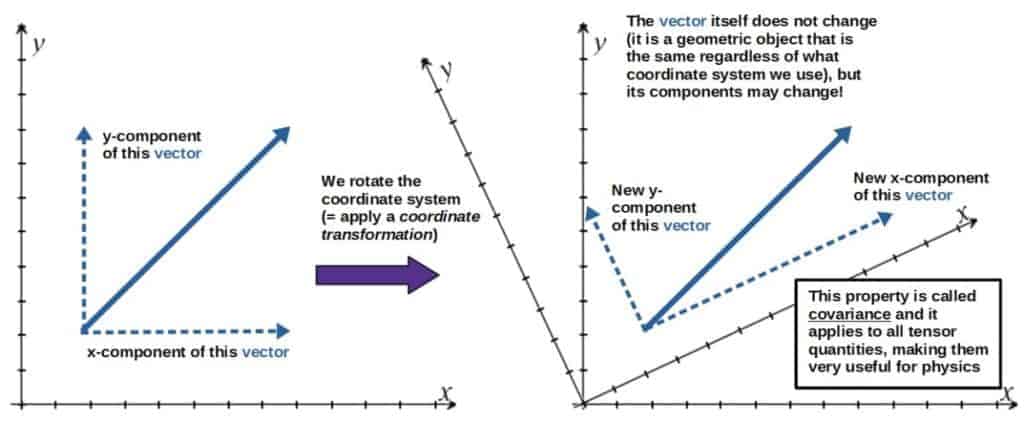
The key property of tensors is that a tensor is always the same in every coordinate system (in a technical sense, we say that a tensor transforms covariantly).

First of all, what is a tensor anyway? A tensor is simply a “collection of objects” (these objects are its tensor components) whose components transform in a nice, predictable way between coordinate changes, while the tensor itself remains unchanged.

A nice intuitive way to understand this is by looking at how a vector behaves under coordinate changes (a vector is, in fact, a tensor of “rank 1”):

Mathematically, the transformation law of the components of a tensor is as follows:

T'_{ij}=\frac{\partial x^m}{\partial x'^i}\frac{\partial x^n}{\partial x'^j}T_{mn}

Note that this is the transformation law for a tensor that has two downstairs indices. A different tensor generally follows the same pattern (there is one of these partial derivatives of the coordinates -terms for each index).

In fact, this often works as the definition of a tensor. So, we can simply define a tensor as any mathematical object whose components transform by the transformation law given above.

Now, a mathematician might say that the definition of a tensor is something more like “a multilinear map from vectors and dual vectors to real numbers”.

If you really want to be accurate and are interested in abstract mathematics, then sure, this definition would be more precise. However, for physics and most physical applications, the definition of a tensor through its transformation properties is perfectly good.

A tensor is typically represented by its components, which we simply write as either downstairs or upstairs indices. These different indices correspond to the different tensor components.

In general relativity, these indices are usually Greek letters and they run from 0 to 3 (the 0-component corresponds to time, while 1, 2 and 3 corresponds to the three spacial directions). In mathematics, it’s typical to use Latin indices, such as i and j.

These tensor components can be nicely represented as a “table” as follows (more accurately, this is called the “matrix representation of a tensor” and it really only works for a two-index tensor, such as the Ricci tensor):

T_{\mu\nu}=\begin{pmatrix}T_{00}&T_{01}&T_{02}&T_{03}\\T_{10}&T_{11}&T_{12}&T_{13}\\T_{20}&T_{21}&T_{22}&T_{23}\\T_{30}&T_{31}&T_{32}&T_{33}\end{pmatrix}

These T’s here are the components of this tensor T_µν. For example, T₀₁ is the component where µ=0 and ν=1.

Now, enough about the general properties of tensors. What we’re really interested in is the Ricci tensor. The Ricci tensor is a tensor (as you may have guessed by now) with two indices, denoted as R_ij (if you’re talking about general relativity, these indices would be Greek letters).

Now, sure, the Ricci tensor is a tensor, but what does it actually represent? Luckily, there is a pretty nice geometric interpretation of the Ricci tensor, which we’ll talk about next.

Geometric Interpretation of The Ricci Tensor

The Ricci tensor is one of the central mathematical objects in the field of differential geometry. This, of course, relates the Ricci tensor directly to geometry in some way. So, what then is the geometric interpretation of the Ricci tensor?

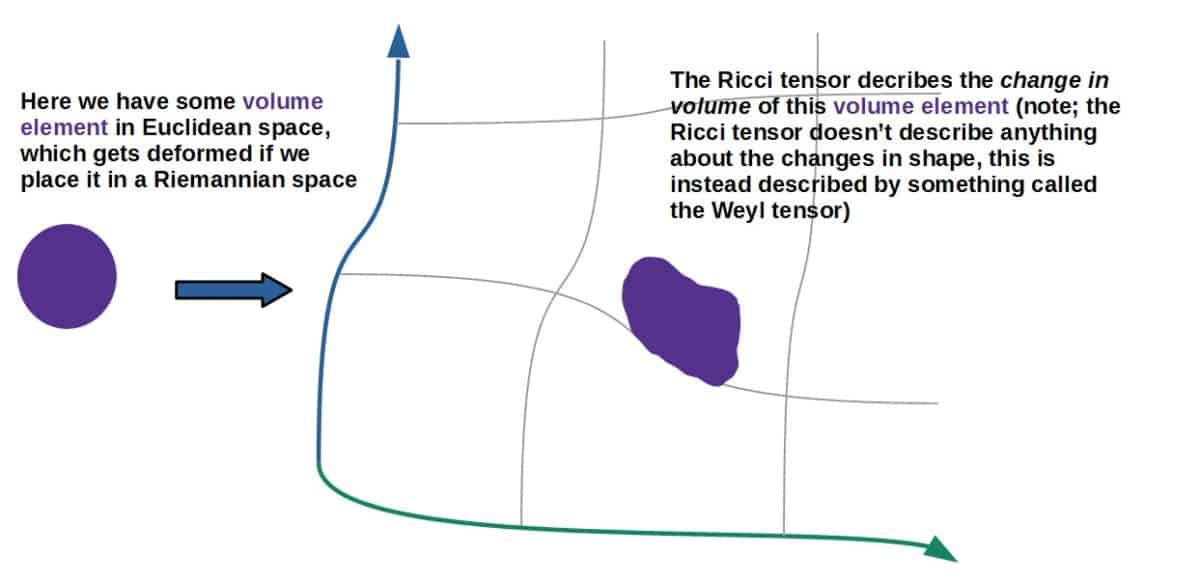
The geometric interpretation of the Ricci tensor is that it describes how much a volume element would differ in curved space compared to Euclidean or flat space. Different components of the Ricci tensor describe how the volume element evolves as one moves along a geodesic in any given direction.

By curved space, I’m essentially referring to a Riemannian manifold, which to put it simply, is just a space in which the basis vectors may vary from place to place (and the geometry of that space is described by a metric tensor).

A nice way to visualize what the Ricci tensor describes is by taking some “volume element” in Euclidean space (this is simply your original Cartesian coordinate system, for example) and imagine placing it in a Riemannian space.

The Ricci tensor would then, in some sense, tell you how much the volume of this volume element changes.

There is a very nice way to actually see why the Ricci tensor describes these volume changes, which is by looking at small differences in the metric of a Riemannian space compared to the metric of a Euclidean space.

This idea can then be extended to volume elements also, and you’ll, in fact, find the Ricci tensor appearing here. The proof can be found below.

Mathematical Proof: Why The Ricci Tensor Describes Volume Changes

Physical Meaning of The Ricci Tensor

Now, the Ricci tensor is useful for describing curvature mathematically, but does it also have a specific physical meaning?

The physical meaning of the Ricci tensor is that it describes how much the spacetime volume of an object changes due to gravitational tides in general relativity. This is because geometrically, the Ricci tensor describes volume changes due to curvature and spacetime curvature is equated to tidal forces.

First of all, to understand all this, let’s think of how the Ricci tensor actually encodes information about changes in spacetime volume.

In general relativity, all objects move through spacetime along geodesics (a geodesic, in a simple sense, is just the “shortest distance between two spacetime points”).

I explain geodesics and really all the important points about general relativity in my introductory article on general relativity.

Now imagine you have two different geodesics (that start parallel to each other).

If the spacetime is curved (in general relativity, this means that there is gravity present), these geodesics may begin deviating from one another.

These geodesics will also, at all times, enclose some kind of volume in spacetime between them.

Geometrically, the Ricci tensor then describes how much this spacetime volume changes as you move along these geodesics. This is actually what we already talked about earlier.

Let’s now think of the physical implications of this. In particular, let’s think of a physical object with some well-defined volume (a ball for example).

In spacetime, all the different parts of the object will follow their own geodesics through spacetime (essentially, you can think of every atom of an object following its own spacetime geodesic).

Therefore, if curvature is present, these geodesics may begin deviating from one another and the spacetime volume between them will change.

Physically, this means that the object will get stretched and squeezed in different directions.

This squeezing and stretching, on the other hand, corresponds to the effect of tidal forces, which are simply the result of geodesic deviation (tidal forces and geodesic deviation is explained in more detail in my general relativity article).

So, the physical description of the Ricci tensor is how much the spacetime volume of an object changes due to gravitational tidal forces.

Also, note that the object gets deformed in spacetime, not just in the usual three-dimensional space, so, in fact, the object will also get stretched or squeezed in time. This effect is called gravitational time dilation.

As a sidenote, I have an article discussing gravitational time dilation near a black hole. The interesting thing about it is that there is actually a subtle geometric explanation of gravitational time dilation that ties really nicely with all the other geometric concepts used in general relativity.

Ricci Tensor In Terms of Christoffel Symbols

Now that we’ve talked about what the Ricci tensor represents, it’s time to discuss some of its properties. In particular, how is the Ricci tensor defined?

Essentially, the Ricci tensor is defined in terms of mathematical objects called Christoffel symbols in the following way:

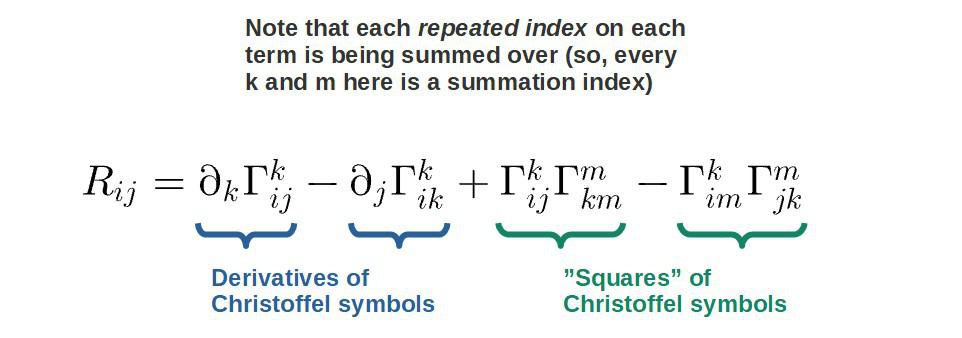
These Christoffel symbols are defined in terms of the metric tensor of a given space and its derivatives:

\Gamma_{ij}^k=\frac{1}{2}g^{km}\left(\partial_jg_{mi}+\partial_ig_{mj}-\partial_mg_{ij}\right)

Now, since the Ricci tensor is built out of the Christoffel symbols (which, on the other hand, is built out of the metric), this means that the Ricci tensor is actually comprised of products of the metric tensor, products of derivatives of the metric and also second derivatives of the metric.

In principle, you could write down the Ricci tensor completely in terms of the metric only, but this would give you a horrendously complicated formula.

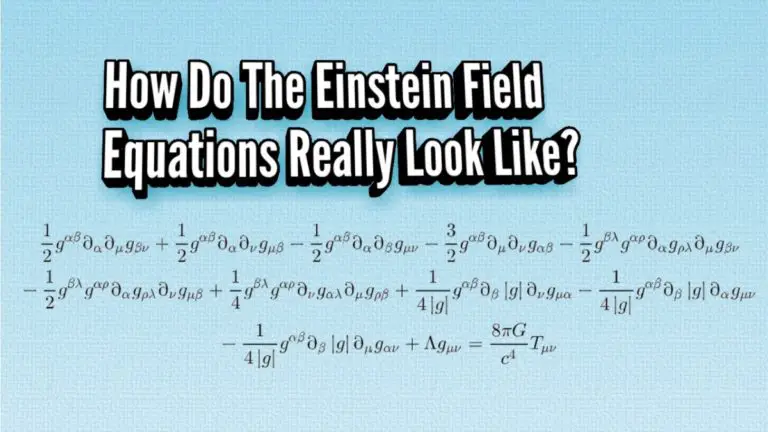
If you want to get a sense of what this looks like, you can check out this page which has the Einstein field equations fully written out in terms of the metric. I also have an entire article on deriving the Einstein field equations in two different ways (which you’ll find here) and actually, both of these derivations rely on the properties of the Ricci tensor.

The much more practical approach is to first calculate the Christoffel symbols through the metric and then based on the properties of the C-symbols, try to simplify the form of the Ricci tensor.

We’ll talk about this and how to calculate the Ricci tensor (as well as some examples) later in the article.

An Intuitive Derivation of The Ricci Tensor

Now, you may wonder why exactly the Ricci tensor is defined in terms of the Christoffel symbols in the way given above. This form can actually be derived by first deriving a tensor called the Riemann tensor and then constructing the Ricci tensor out of that.

However, to derive this will require a decent bit of tensor calculus knowledge, so if you’re not familiar with it, you may want to skip the derivation given below.

Derivation of the Ricci Tensor From Parallel Transport (click to see more)

Ricci Tensor Symmetries

The Ricci tensor has an important symmetry, which is that you can interchange its two indices freely:

R_{\mu\nu}=R_{\nu\mu}

Note; I’m using spacetime indices here, simply because I think they look cooler. Using Greek as opposed to Latin indices, however, makes no difference.

Fundamentally, this symmetry comes from the fact that the Christoffel symbols which the Ricci tensor is built out of are by definition symmetric. This is because Riemannian geometry (and general relativity) are known as torsion-free theories.

Why Is The Ricci Tensor Symmetric? (A Simple Proof)

Now, the symmetry of the Ricci tensor comes from the fact that in Riemannian geometry, an object known as the torsion tensor is zero (this is the assumption of a “torsion-free connection”):

T_{\mu\nu}^{\lambda}=\Gamma_{\mu\nu}^{\lambda}-\Gamma_{\nu\mu}^{\lambda}=0

This, on the other hand, implies that the Christoffel symbols have to be symmetric on the two lower indices:

\Gamma_{\mu\nu}^{\lambda}=\Gamma_{\nu\mu}^{\lambda}

Also, the metric tensor itself is by assumption symmetric (this is more or less a convention, but a useful one).

The two above facts are actually enough to prove that the Ricci tensor is indeed symmetric. You’ll find a simple proof of this below.

Derivation of the Ricci Tensor From Parallel Transport

How To Calculate The Ricci Tensor (Step-By-Step)

In this section, I’ll go over some of the more practical stuff in regards to actually calculating the Ricci tensor for different situations. What the Ricci tensor looks like in any given space is ultimately determined by what the metric is in that given space.

The general steps for calculating the Ricci tensor are as follows:

1. Specify a metric tensor (either in matrix form or the line element of the metric).
2. Calculate the Christoffel symbols from the metric.
3. Calculate the components of the Ricci tensor from the Christoffel symbols.
4. Special case: in general relativity, if the Ricci scalar for a given spacetime is zero, it’s possible to calculate the Ricci tensor directly from the energy-momentum tensor (without the Christoffel symbols).

Now, even though generally, the Ricci tensor has quite a complicated form, once you’ve specified a given metric and the Christoffel symbols in terms of that metric, the complexity of the Ricci tensor can usually be simplified a lot.

Also, there is an extremely efficient (and to my surprise, not very commonly used) method to calculate the Christoffel symbols, which can save you a lot of time in many computations. I discuss the method in this article.

Calculating Ricci Tensor From The Metric

Calculating the Ricci tensor from a given metric essentially follows the exact steps specified above. Down below I’ve included a bunch of examples of different Ricci tensors and the calculations of these all follow exactly the steps given above.

Also, for the special case mentioned in the above steps, you can find an example down below for the Reissner-Nordström metric (which describes charged black holes in general relativity).

The real beauty of this special case is that if the Ricci scalar happens to be zero, then the Einstein field equations of general relativity essentially reduce to just an equation for the Ricci tensor:

R_{\mu\nu}-\frac{1}{2}Rg_{\mu\nu}=\frac{8\pi G}{c^4}T_{\mu\nu}\ \ \Rightarrow\ \ R_{\mu\nu}=\frac{8\pi G}{c^4}T_{\mu\nu}

This is particularly useful because it allows us to essentially skip the whole process of calculating the Christoffel symbols. All we have to specify is the energy-momentum tensor, which usually has a fairly simple form.

Examples of Ricci Tensors

Below I’ve collected some of the most commonly used Ricci tensors as well as where they come from. Keep in mind that the point of this article is not to derive these, so I haven’t included the explicit calculations here.

But, of course, you can do them yourself if you really wish to with the help of all the information, formulas and instructions found earlier in this article.

You can also find a comprehensive list of different Christoffel symbols for various metrics in this article.

Ricci Tensor In Flat Space

The first particularly simple example is the Ricci tensor in flat space. In flat space, the Ricci tensor is zero:

R_{\mu\nu}=0

Moreover, a tensor being equal to zero means that each of its components has to be zero, so in matrix form, this says:

R_{\mu\nu}=\begin{pmatrix}0&0&0&0\\0&0&0&0\\0&0&0&0\\0&0&0&0\end{pmatrix}

Now, a key point here is that the Ricci tensor being zero technically does not imply that the space has to be completely flat. It only has to be what is called Ricci-flat (which is defined as R_µν=0).

It is true that a flat space does also have R_µν=0, however, it is not a sufficient condition. If the Riemann tensor, on the other hand, is zero, then the space is definitely flat.

Ricci Tensor of a Sphere

This example is the Ricci tensor on the surface of a 3-dimensional sphere.

Now, since the surface itself is basically a 2-dimensional space, the metric and the Ricci tensor are therefore both 2×2-matrices (this is enough to specify the space on the surface). The surface is sometimes called a 2-sphere, which really just means the surface of a 3D sphere.

Now, the surface of this sphere is defined by the fact that the distance (radius r) from the center is a constant. The two coordinates needed to specify a point on the surface are two angles, θ and φ.

The line element on this sphere (the 2-sphere) has the form:

ds^2=r^2d\theta^2+r^2\sin^2\theta d\phi^2

The metric can then be written as a 2×2-matrix:

g_{ij}=\begin{pmatrix}r^2&0\\0&r^2\sin^2\theta\end{pmatrix}

Note that these latin indices (i and j) are typically used to refer to spacial components only, while greek indices refer to spacetime components (this example, of course, does not involve time at all).

The Christoffel symbols calculated from this metric are then:

\Gamma_{ij}^1=\begin{pmatrix}0&0\\0&-\sin\theta\cos\theta\end{pmatrix}\\\Gamma_{ij}^2=\begin{pmatrix}0&\cot\theta\\\cot\theta&0\end{pmatrix}

The Ricci tensor then takes on a very simple explicit form:

R_{ij}=\frac{g_{ij}}{r^2}

Since the metric has two components, the Ricci tensor does as well. We can collect these components into a nice 2×2-matrix (just calculate the components from the above form by plugging in the metric):

R_{ij}=\begin{pmatrix}1&0\\0&\sin^2\theta\end{pmatrix}

Ricci Tensor For The Schwarzschild Metric

The Schwarzschild metric is a solution of Einstein’s field equations in a vacuum. In particular, it described the spacetime around a spherically symmetric mass.

Now, since it is a vacuum solution, the energy-momentum tensor on the right-hand side of Einstein’s equations is zero (you can read my introductory general relativity article for more on the Einstein field equations). This then actually corresponds to the Ricci tensor being zero as well:

R_{\mu\nu}=0

So, a particularly nice condition for a vacuum is that R_µν=0. This, of course, doesn’t mean that the spacetime is flat (since the Riemann tensor isn’t zero in this case), so there is definitely gravity present in the Schwarzschild spacetime.

Another example of a vacuum Ricci tensor (R_µν=0) is the Ricci tensor for the Kerr metric. This describes the spacetime and gravity outside a rotating spherically symmetric mass (instead of the Schwarzschild solution, which only describes a stationary mass).

Ricci Tensor For The Robertson-Walker (FRW) Metric

The Robertson-Walker metric (usually just called the FRW metric) describes what is called a maximally symmetric spacetime (one that is both homogeneous and isotropic).

This metric can, for example, describe our universe on a large scale and it, in fact, predicts the expansion of the universe.

I discuss the FRW metric, its associated Friedmann equations and their predictions (such as the expansion of the universe) more in this article.

The line element for this metric is:

ds^2=-dt^2+\frac{a^2\left(t\right)}{1-kr^2}dr^2+a^2\left(t\right)r^2d\theta^2+a^2\left(t\right)r^2\sin^2\theta d\phi^2

Here, k is a curvature parameter that can take on the values -1, 0 or 1, depending on the geometry of the spacetime (to describe a flat universe, k=0, for example). The a(t)-parameter is called the scale factor and it is a function of time that holds information about the expansion of the universe (or whatever space this metric happens to be describing).

This metric has the matrix form:

g_{\mu\nu}=\begin{pmatrix}-1&0&0&0\\0&\frac{a^2}{1-kr^2}&0&0\\0&0&a^2r^2&0\\0&0&0&a^2r^2\sin^2\theta\end{pmatrix}

For this metric, there are 19 Christoffel symbols in total (which are not all independent). These can be written as four different matrices with the upper index labeling which of the four matrices we’re talking about:

\Gamma_{\mu\nu}^0=\begin{pmatrix}0&0&0&0\\0&\frac{a\dot{a}}{c\left(1-kr^2\right)}&0&0\\0&0&\frac{1}{c}a\dot{a}r^2&0\\0&0&0&\frac{1}{c}a\dot{a}r^2\sin^2\theta\end{pmatrix}

Here, dot{a} denotes da/dt (a being a function of time) and c is the speed of light.

\Gamma_{\mu\nu}^1=\begin{pmatrix}0&\frac{\dot{a}}{ca}&0&0\\\frac{\dot{a}}{ca}&\frac{kr}{1-kr^2}&0&0\\0&0&-r\left(1-kr^2\right)&0\\0&0&0&-r\sin^2\theta\left(1-kr^2\right)\end{pmatrix}\\\Gamma_{\mu\nu}^2=\begin{pmatrix}0&0&\frac{\dot{a}}{ca}&0\\0&0&\frac{1}{r}&0\\\frac{\dot{a}}{ca}&\frac{1}{r}&0&0\\0&0&0&-\sin\theta\cos\theta\end{pmatrix}\\\Gamma_{\mu\nu}^3=\begin{pmatrix}0&0&0&\frac{\dot{a}}{ca}\\0&0&0&\frac{1}{r}\\0&0&0&\cot\theta\\\frac{\dot{a}}{ca}&\frac{1}{r}&\cot\theta&0\end{pmatrix}

The Ricci tensor for this metric has four components, with the time component being:

R_{00}=-\frac{3}{c^2}\frac{\ddot a}{a}

Here, a with two dots means the second time derivative of a(t), d²a/dt² (“acceleration”).

The space components are given by:

R_{ij}=\left(a\ddot a+2\dot a^2+2kc^2\right)\frac{g_{ij}}{a^2}

We can collect all of these into a 4×4-matrix:

R_{\mu\nu}=\begin{pmatrix}-\frac{3}{c^2}\frac{\ddot a}{a}&0&0&0\\0&\frac{a\ddot a+2\dot a^2+kc^2}{1-kr^2}&0&0\\0&0&\left(a\ddot a+2\dot a^2+kc^2\right)r^2&0\\0&0&0&\left(a\ddot a+2\dot a^2+kc^2\right)r^2\sin^2\theta\end{pmatrix}

Ricci Tensor For The Reissner-Nordström Metric

An interesting and more complicated black hole metric is the Reissner-Nordström metric. This describes a charged (but non-rotating) black hole or whatever other spherically symmetric mass. This spacetime is basically identical to the Schwarzschild spacetime, except that the black hole is now charged.

The metric is given in matrix form by:

g_{\mu\nu}=\begin{pmatrix}-\left(1-\frac{r_s}{r}+\frac{r_Q^2}{r^2}\right)&0&0&0\\0&\frac{1}{1-\frac{r_s}{r}+\frac{r_Q^2}{r^2}}&0&0\\0&0&r^2&0\\0&0&0&r^2\sin^2\theta\end{pmatrix}

The two parameters here are given by:

r_Q^2=\frac{Q^2G}{4\pi\varepsilon_0c^4}\ \ \ \&\ \ \ \ r_s=\frac{2GM}{c^2}

Here, Q and M are the charge and mass of the black hole, c is the speed of light, G is the gravitational constant and ε₀ is the electric constant (also called vacuum permittivity).

Since the black hole is charged, there is an electric field around it and therefore, the energy-momentum tensor is not zero. This means that the right-hand side of the Einstein field equations is not zero and so, the Ricci tensor is not zero either.

In fact, it can be shown that the Ricci scalar for this metric is zero, so the Einstein equations have the form:

R_{\mu\nu}=8\pi GT_{\mu\nu}

From this, it’s possible to directly calculate the Ricci tensor without even needing any of the Christoffel symbols. The energy-momentum tensor (for an electromagnetic field) is given in terms of the EM field tensor F_µν:

T_{\mu\nu}=\frac{1}{\mu_0}\left(F_{\mu\beta}F_{\nu\alpha}g^{\alpha\beta}-\frac{1}{4}g_{\mu\nu}F_{\alpha\beta}F^{\alpha\beta}\right)

For a Reissner-Nordström black hole, it only has one component of the EM tensor, namely a radial electric field:

E=\frac{Q}{4\pi\varepsilon_0r^2}

This electric field corresponds to the 01-component of the EM tensor. If we take this to be the only component of the EM tensor, then the energy-momentum tensor has the form:

T_{\mu\nu}=\frac{1}{\mu_0}\left(F_{\mu0}F_{\nu0}g^{00}+F_{\mu1}F_{\nu1}g^{11}\right)+\frac{Q^2c^2}{32\pi^2\varepsilon_0r^4}g_{\mu\nu}

When you plug this into the Einstein field equations, you’ll find that the Ricci tensor actually takes on quite a simple form:

R_{\mu\nu}=\mp\frac{r_Q^2}{r^4}g_{\mu\nu}

The notation here may seem a bit awkward, but this is only because the Ricci tensor has a different signature than the metric for the R₀₀ and R₁₁ -components. Namely, these first two components have a minus sign and the last two (R₂₂ and R₃₃) have a plus sign. This minus-plus sign in there is just to indicate this fact.

Explicitly, you could write them as:

R_{00}=-\frac{r_Q^2}{r^4}g_{00}\ {,}\ R_{11}=-\frac{r_Q^2}{r^4}g_{11}\ {,}\ R_{22}=\frac{r_Q^2}{r^4}g_{22}\ {,}\ R_{33}=\frac{r_Q^2}{r^4}g_{33}

Anyway, plugging in the metric into these and simplifying a bit, we can express the Ricci tensor as a 4×4-matrix, as usual:

R_{\mu\nu}=\begin{pmatrix}\frac{r_Q^2}{r^4}-\frac{r_Q^2r_s}{r^5}+\frac{r_Q^4}{r^6}&0&0&0\\0&\frac{r_Q^2}{r^3r_s-r^4-r^2r_Q^2}&0&0\\0&0&\frac{r_Q^2}{r^2}&0\\0&0&0&\frac{r_Q^2}{r^2}\sin^2\theta\end{pmatrix}