How Does Gravity Work Underground? (An In-Depth Explanation)

Gravity is a force that pulls objects down and it is commonly known to be approximately constant on the surface of Earth. What would happen if you, however, dug a deep hole into the Earth? Would the gravity you experience change as you went underground and how would it change with depth?

In short, gravity certainly exists underground, but it decreases as you go deeper. In an idealized case, gravity would decrease linearly with depth, however, a more realistic description of gravity underground is given by the PREM model, according to which gravity is at its strongest at the boundary of the outer core.

In this article, we’ll take a look at how gravity can be modeled underground as well as how it actually changes as you go deeper. At the end, we’ll also talk about how going underground would practically affect the weight you feel (kind of similar to the idea that you’d feel lighter on the surface of the Moon).

How Does Gravity Change With Depth? (Mathematical Models Included)

If you know a little bit about physics, at a glance you may think that the force of gravity would actually get larger as you go deeper underground (closer to the center of Earth). You may think this way if you just look at Newton’s law of gravity (which describes the gravitational force F an object of mass m would experience):

$F=\frac{GMm}{r^2}$

Here M is the mass of the Earth, G is the gravitational constant and r is the distance from Earth’s center.

For this case, it will be more useful to only look at the gravitational acceleration g and not the force itself (because the force depends on your mass too, which we don’t really care about right now). That we can get by the simple fact that F=ma (or F=mg, since the gravitational acceleration is denoted by g):

$mg=\frac{GMm}{r^2}\ \ \Rightarrow\ \ g=\frac{GM}{r^2}$

Based on this, if you make r (distance from the center of the Earth) smaller by going underground, gravity (gravitational acceleration) would get larger. This is, however, not actually correct.

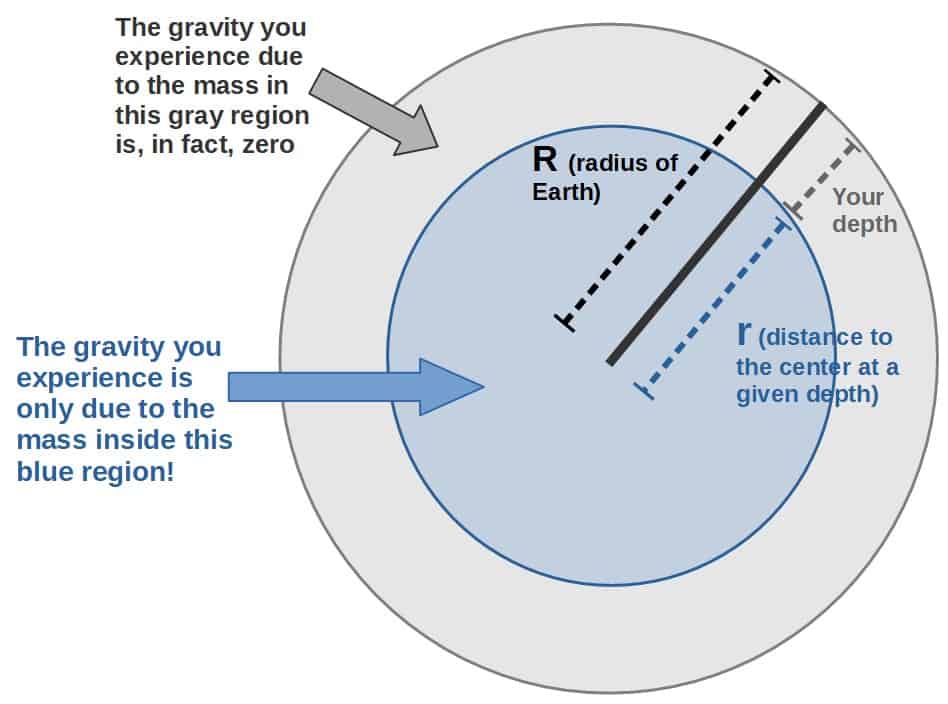
The problem is that, actually, the gravity you’d experience is only due to the mass of the Earth that is inside the given radius r (picture below), not any of the mass outside this radius. This means that if you go underground, there is actually less mass pulling you down, but at the same time, the radius r is smaller. So, is the gravity therefore stronger or weaker?

Now, there are two mathematical models that we’ll take a look at in this article (don’t worry, I’ll explain these in detail soon):

• An idealized model, which only uses the average density of Earth (this formula is derived directly from Gauss’s law):

$g\left(r\right)=\frac{4}{3}\pi G\rho r$

• A more realistic model, which takes into account the fact that the density of Earth actually varies from place to place (this formula is based on the PREM model):

$g\left(r\right)=\begin{cases}\sum_{n=0}^i\alpha_nr^n&{,}\ 0\le r<r_{\max}\\\sum_{n=0}^j\beta_nr^n&{,}\ r_{\max}\le r\le R\end{cases}$

Here, the α’s and β’s are some suitable coefficients that fit the data given by the PREM model (you’ll find these coefficients later in the article).

The notable thing here for now is that both of these models describe the gravitational acceleration at a given (underground) distance r from the center of Earth (or if you wish to express it in terms of the depth underground d, just insert r=R-d).

The first formula increases linearly as you increase the distance r, but the second one actually changes as a polynomial with regards to r. Later, we’ll take a look at what each of these coefficients and symbols in the formula actually mean and the results should be quite surprising and interesting.

Gravitational Acceleration Underground Using Gauss’s Law (Simple Formula)

The first formula we’ll look at is the following (the derivation of this can be found down below):

$g\left(r\right)=\frac{4}{3}\pi G\rho r$

The values in the formula are the following; r is the distance from the center of Earth (this works only for r<R), G is the gravitational constant (G=6.674×10^-11 m³/(kg·s²)) and ρ is the average density of Earth (ρ=5510 kg/m³).

Derivation Using Gauss's Law For Gravity (click to see more)

Now, this formula is really only an estimate and it isn’t perfectly correct. The inaccuracies in the model mainly come from two simplifications that, in reality, are not really true:

1. It assumes that the Earth is perfectly spherical (shaped like a perfect ball), which is not quite correct (although not massively wrong either).
2. It uses the average density of Earth, but does not take into account the fact that the density is actually different at different layers of the Earth. For example, the density of the liquid outer core of the Earth is about 9900 kg/m³, while the density at the Earth’s surface (crust) is only around 2200 kg/m³ (source: a USGS publication, which is based on data from D.L. Anderson’s book “Theory of the Earth”).

For our purposes, however, it may be useful to look at the above formula as a function of depth from the Earth’s surface (d) rather than the distance from the center (r). The distance r can be expressed as simply the radius of Earth (R) minus the depth (d), i.e. r=R-d.

From this, we get the gravitational acceleration as a function of depth:

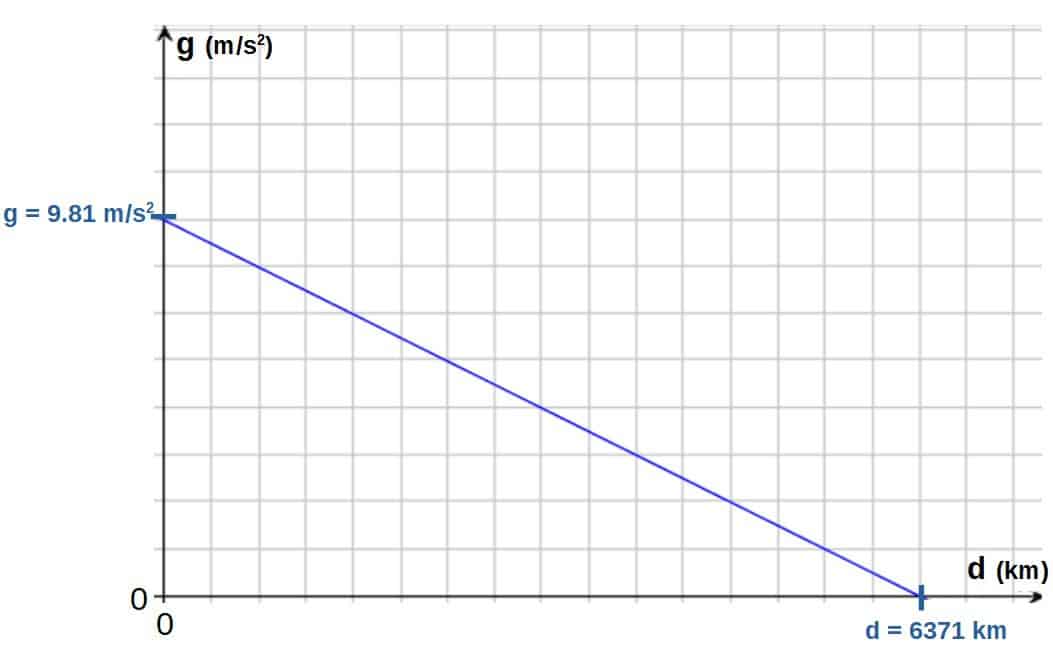
$g\left(d\right)=\frac{4}{3}\pi G\rho\left(R-d\right)$

If we plug in all the values (R=6371 km and for the other values, see above), we can easily calculate the strength of gravity at different depths by using this function. Down below you’ll find a table of some values as well as a graph.

Depth (km)	Gravitational acceleration g (m/s2)
0	9.81
10	9.80
50	9.74
100	9.66
1000	8.27
3000	5.19
6371 (Earth’s radius)	0

This model, as stated above, is really only an idealization and once you take into account the variation in Earth’s density as well, the results change quite a bit and become a little more complicated. The results, however, are very interesting and that is what we’ll look at next.

Gravitational Acceleration Underground According To The PREM model

For this, we will be using a much more realistic theory of the different layers of the Earth, which is called the Preliminary Reference Earth Model (PREM). This model aims to describe different aspects of Earth at the different layers of Earth or in other words, as a function of radius.

One set of data that this model describes is the gravitational acceleration at any given radius (for this article, I have used the data from a 1981 paper by A.M. Dziewonski and D.L. Anderson, which you’ll find the data in a nice downloadable format here).

What you’ll actually find is that there is a point below the Earth’s surface where gravity is at its maximum and that the gravitational acceleration actually varies as a polynomial function of r rather than a linear one as follows:

$g\left(r\right)=\begin{cases}\sum_{n=0}^i\alpha_nr^n&{,}\ 0\le r<r_{\max}\\\sum_{n=0}^j\beta_nr^n&{,}\ r_{\max}\le r\le R\end{cases}$

Now, if we fit in the data from the PREM model, we find that the function looks as follows (where this comes from is explained down below):

$g\left(r\right)=\begin{cases}\alpha_0+\alpha_1r+\alpha_2r^2&{,}\ 0\le r<r_{\max}\\\beta_0+\beta_1r+\beta_2r^2+\beta_3r^3+\beta_4r^4+\beta_5r^5&{,}\ r_{\max}\le r\le R\end{cases}$

Here, r_max is the value of r where the gravitational acceleration is at its strongest (about 3470 km) and R is the radius of the Earth (6371 km).

The coefficients are given in the table below:

Coefficient	Numerical value
α0	-1.942×10-2
α1	3.853×10-3
α2	−2.22×10-7
β0	16.82
β1	3.114×10-3
β2	-4.059×10-6
β3	1.149×10-9
β4	-1.278×10-13
β5	4.894×10-18

Where Does This Function Come From? (click to see more)

Now, the notable thing about the above function is that it is a polynomial with respect to r. In other words, it is not just a simple linear function, but it actually has a maximum value at about 3470 km (see the graph below).

This corresponds to a gravitational acceleration of about 10.7 m/s² (which is comparable to the gravity at the surface of Saturn!). For comparison, the gravitational acceleration at Earth’s surface is 9.81 m/s².

Now, you may ask; why does the gravitational acceleration actually vary like this? Why is it at its maximum at 3470 km from the center of Earth? The answer comes from looking at the different densities of each layer of Earth.

Now, if you actually think about what affects gravity, it is only the mass inside a certain volume (this is what Gauss’s law essentially tells us), which practically just means that density has to be taken into account as well (since there is more mass inside certain layers of Earth than inside others).

The most dense layers of Earth actually happen to be the outer and inner core. Not surprisingly, at the distance 3470 km from Earth’s center is actually the boundary between the mantle and the outer core (this boundary is called the Gutenberg discontinuity).

If you were standing at this boundary, the most dense layers of Earth (outer and inner core) would be right beneath your feet (see the picture below). You can then imagine how it makes sense that gravity is at its strongest right at this boundary.

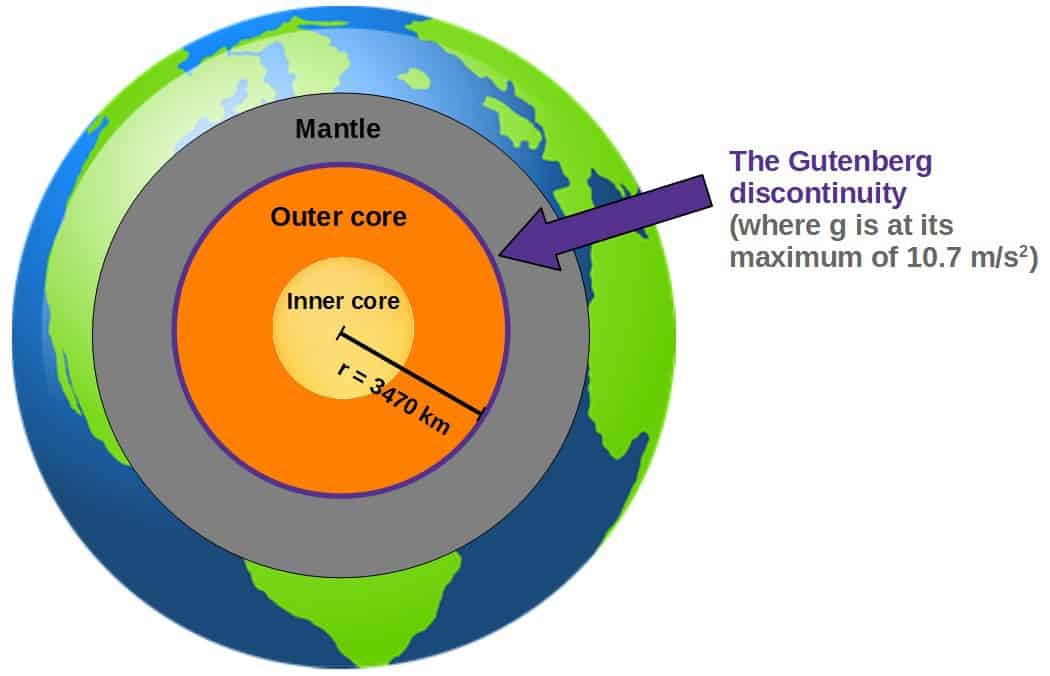
If you go upwards from this boundary, gravity would get weaker as you approach the surface of Earth, which is due to the non-uniformly distributed mass of the Earth.

If you were to go deeper, there would be less of this really dense material (the outer and inner core) actually affecting the gravity you feel, so gravity should then get weaker also.

Thus, the distance of 3470 km from Earth’s center is where gravity is at its strongest (or equivalently, a depth of about 2900 km as measured from the Earth’s surface), which is exactly what the graph from the PREM model data tells us as well (see the graph from earlier).

How Would Your Weight Change If You Went Underground (& Why)?

An interesting question regarding how gravity works underground is whether you’d actually weigh less underground.

There are two ways to look at this, which I’ll answer in this section:

• Would your weight be different underground and how? In other words, what number would a weight scale show underground compared to what it would show at the surface of Earth?
• Would you feel lighter or heavier underground? In other words, would there be a similar effect as, for example, there is on the moon where you could jump significantly higher.

How Would Your Weight Be Different Underground?

Weight is essentially the measure of the gravitational force exerted on you by the Earth, in this case (notice that it is different from mass; if you wish, you can read more about the differences of these from this Wikipedia article).

Therefore, since the gravitational force (or gravitational acceleration, equivalently) is different underground, your weight would indeed be different underground as well.

In fact, your weight would be the greatest at the boundary between the mantle and the outer core (where the distance from the center of Earth is about 3470 km), where the gravitational acceleration is about 10.7 m/s² (see earlier if you missed where this comes from).

Now, the interesting question is; how is your weight actually different? Would a weight scale, for example, show a different number underground?

The answer is that it indeed would. As an example, say your mass is 70 kg (measured with a weight scale at the surface).

Now say you’re at the point of maximum gravity (3470 km from the center). If you were to now measure your mass with the same scale, it would show about 76 kg instead of 70 kg. That’s an 8% difference!

How Is This Calculated? (click to see more)

Now, this of course, does not mean that your mass would actually be different. The discrepancy here comes from the fact that weight scales measure mass by measuring the gravitational force, not directly mass itself.

The number you’d see will depend on the scale you use as well. Most electronic scales would indeed show this difference, but there are also scales which are not affected by differences in gravity (such as balancing scales).

Also, close to the center of Earth, your weight would be quite small and as you get closer and closer to the center, your weight would indeed go to zero. Thus, at the center of the Earth, you’d actually be weightless.

Would You Actually Feel Heavier or Lighter Underground?

Another quite interesting question is whether you would actually feel heavier or lighter underground, below the surface of the Earth. The answer is that, you would indeed feel different.

First of all, what do I mean by the word “feel”? By this, I simply mean some measure of easy it would be to move around. For example the stronger the gravity, the harder it would be to lift your feet or jump up, so you’d feel much heavier.

Now, whether you’d feel heavier of lighter will, of course, depend on where you are underground. If you are at the point of maximum gravity (at a distance of 3470 km from Earth’s center), you would feel heavier.

At this depth, it would be more difficult to jump up, for example, and you’d fall back down a lot faster. This is simply due to the fact that you’re being pulled down by a stronger force of gravity.

If, on the other hand, you are close to the center of the Earth where gravity is approximately zero, you would feel weightless and you’d be able to float (just like you could if you were in space).

So, the bottom line is that, yes, your weight would be different underground. Whether it would be more or less than at the surface, will depend on what the gravitational acceleration at that particular depth is.